Tutorial: Detection / recognition of multiple rectangles and extracting with OpenCV
Categories Computer Vision, Uncategorized
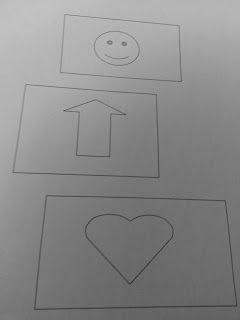
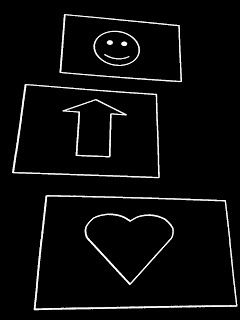
This tutorial will be focused on being able to take a picture and extract the rectangles in the image that are above a certain size:
I am using OpenCV 2.4.2 on Microsoft Visual Express 2008 but it should work with other version as well.
Thanks to: opencv-code.com for their helpful guides
Step 1: Clean up
So once again, we’ll use my favourite snippet for cleaning up an image:
Apply a Gaussian blur and using an adaptive threshold for binarzing the image
//Apply blur to smooth edges and use adapative thresholding cv::Size size(3,3); cv::GaussianBlur(img,img,size,0); adaptiveThreshold(img, img,255,CV_ADAPTIVE_THRESH_MEAN_C, CV_THRESH_BINARY,75,10); cv::bitwise_not(img, img);
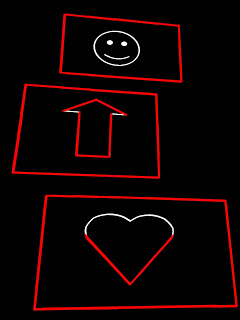
Step 2: Hough Line detection
Use a probabilistic Hough line detection to figure out where the lines are. This algorithm works by going through every point in the image and checking every angle.
vector<Vec4i> lines; HoughLinesP(img, lines, 1, CV_PI/180, 80, 100, 10);
And here we have the results of the algorithm:
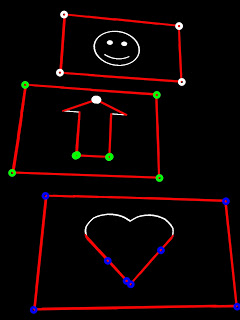
Step 3: Use connected components to determine what they shapes are
This is the most complex part of the algorithm (general pseudocode):
First, initialize every line to be in an undefined group
For every line compute the intersection of the two line segments (if they do not intersect ignore the point)
If both lines are undefined, make a new group out of them
If only one line is defined in a group, add the other line into the group.
If both lines are defined than add all the lines from one group into the other group
If both lines are in the same group, do nothing
Intersection function: (modified from: http://opencv-code.com/tutorials/automatic-perspective-correction-for-quadrilateral-objects/)
cv::Point2f computeIntersect(cv::Vec4i a, cv::Vec4i b)
{
int x1 = a[0], y1 = a[1], x2 = a[2], y2 = a[3];
int x3 = b[0], y3 = b[1], x4 = b[2], y4 = b[3];
if (float d = ((float)(x1-x2) * (y3-y4)) - ((y1-y2) * (x3-x4)))
{
cv::Point2f pt;
pt.x = ((x1*y2 - y1*x2) * (x3-x4) - (x1-x2) * (x3*y4 - y3*x4)) / d;
pt.y = ((x1*y2 - y1*x2) * (y3-y4) - (y1-y2) * (x3*y4 - y3*x4)) / d;
//-10 is a threshold, the POI can be off by at most 10 pixels
if(pt.x<min(x1,x2)-10||pt.x>max(x1,x2)+10||pt.y<min(y1,y2)-10||pt.y>max(y1,y2)+10){
return Point2f(-1,-1);
}
if(pt.x<min(x3,x4)-10||pt.x>max(x3,x4)+10||pt.y<min(y3,y4)-10||pt.y>max(y3,y4)+10){
return Point2f(-1,-1);
}
return pt;
}
else
return cv::Point2f(-1, -1);
}
Connected components
int* poly = new int[lines.size()];
for(int i=0;i<lines.size();i++)poly[i] = - 1;
int curPoly = 0;
vector<vector<cv::Point2f> > corners;
for (int i = 0; i < lines.size(); i++)
{
for (int j = i+1; j < lines.size(); j++)
{
cv::Point2f pt = computeIntersect(lines[i], lines[j]);
if (pt.x >= 0 && pt.y >= 0&&pt.x<img2.size().width&&pt.y<img2.size().height){
if(poly[i]==-1&&poly[j] == -1){
vector<Point2f> v;
v.push_back(pt);
corners.push_back(v);
poly[i] = curPoly;
poly[j] = curPoly;
curPoly++;
continue;
}
if(poly[i]==-1&&poly[j]>=0){
corners[poly[j]].push_back(pt);
poly[i] = poly[j];
continue;
}
if(poly[i]>=0&&poly[j]==-1){
corners[poly[i]].push_back(pt);
poly[j] = poly[i];
continue;
}
if(poly[i]>=0&&poly[j]>=0){
if(poly[i]==poly[j]){
corners[poly[i]].push_back(pt);
continue;
}
for(int k=0;k<corners[poly[j]].size();k++){
corners[poly[i]].push_back(corners[poly[j]][k]);
}
corners[poly[j]].clear();
poly[j] = poly[i];
continue;
}
}
}
}
The circles represent the points of intersection and the colours represent the different shapes.
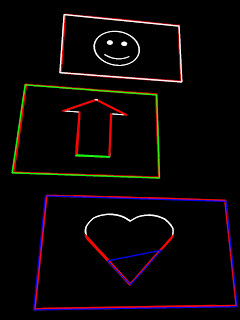
Step 4: Find corners of the polygon
Now we need to find corners of the polygons to get the polygon formed from the point of intersections.
Pseudocode:
For each group of points:
Compute mass center (average of points)
For each point that is above the mass center, add to top list
For each point that is below the mass center, add to bottom list
Sort top list and bottom list by x val
first element of top list is left most (top left point)
last element of top list is right most (top right point)
first element of bottom list is left most (bottom left point)
last element of bottom list is right most (bottom right point)
bool comparator(Point2f a,Point2f b){
return a.x<b.x;
}
void sortCorners(std::vector<cv::Point2f>& corners, cv::Point2f center)
{
std::vector<cv::Point2f> top, bot;
for (int i = 0; i < corners.size(); i++)
{
if (corners[i].y < center.y)
top.push_back(corners[i]);
else
bot.push_back(corners[i]);
}
sort(top.begin(),top.end(),comparator);
sort(bot.begin(),bot.end(),comparator);
cv::Point2f tl = top[0];
cv::Point2f tr = top[top.size()-1];
cv::Point2f bl = bot[0];
cv::Point2f br = bot[bot.size()-1];
corners.clear();
corners.push_back(tl);
corners.push_back(tr);
corners.push_back(br);
corners.push_back(bl);
}
for(int i=0;i<corners.size();i++){
cv::Point2f center(0,0);
if(corners[i].size()<4)continue;
for(int j=0;j<corners[i].size();j++){
center += corners[i][j];
}
center *= (1. / corners[i].size());
sortCorners(corners[i], center);
}
Step 5: Extraction
The final step is extract each rectangle from the image. We can do this quite easily with the perspective transform from OpenCV. To get an estimate of the dimensions of the rectangle we can use a bounding rectangle of the corners. If the dimensions of that rectangle are under our wanted area, we ignore the polygon. If the polygon also has less than 4 points we can ignore it as well.
for(int i=0;i<corners.size();i++){
if(corners[i].size()<4)continue;
Rect r = boundingRect(corners[i]);
if(r.area()<50000)continue;
cout<<r.area()<<endl;
// Define the destination image
cv::Mat quad = cv::Mat::zeros(r.height, r.width, CV_8UC3);
// Corners of the destination image
std::vector<cv::Point2f> quad_pts;
quad_pts.push_back(cv::Point2f(0, 0));
quad_pts.push_back(cv::Point2f(quad.cols, 0));
quad_pts.push_back(cv::Point2f(quad.cols, quad.rows));
quad_pts.push_back(cv::Point2f(0, quad.rows));
// Get transformation matrix
cv::Mat transmtx = cv::getPerspectiveTransform(corners[i], quad_pts);
// Apply perspective transformation
cv::warpPerspective(img3, quad, transmtx, quad.size());
stringstream ss;
ss<<i<<".jpg";
imshow(ss.str(), quad);
}